Using the Mean Value Theorem
Samuel Baker
6 min read
Listen to this study note
Study Guide Overview
This study guide covers the Mean Value Theorem (MVT). It explains the theorem, its conditions (continuity and differentiability), and its formula. It includes a walkthrough example and practice problems applying the MVT to find a point c where the instantaneous rate of change equals the average rate of change over a given interval. The guide also reviews the concepts of continuity and differentiability.
#5.1 Using the Mean Value Theorem
In the previous unit, we learned all about applying derivatives to different real-world contexts. What else are derivatives useful for? Turns out, we can also use derivatives to determine and analyze the behaviors of functions! 👀
#📈 Mean Value Theorem
The Mean Value Theorem states that if a function f is continuous over the interval and differentiable over the interval , then there exists a point within that open interval where the instantaneous rate of change of the function at equals the average rate of change of the function over the interval .
In other words, if a function f is continuous over the interval and differentiable over the interval , there exists some on such that .
!Untitled
Visual representation of mean value theorem
Image Courtesy of Sumi Vora and Ethan Bilderbeek
Yet another way to phrase this theorem is that if the stated conditions of continuity and differentiability are satisfied, there is a point where the slope of the tangent line is equivalent to the slope of the secant line between and .
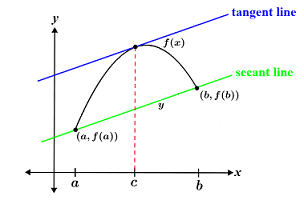
Graph depicting the Mean Value Theorem
Image Courtesy of Math.net
Remember from Unit 1, to be continuous over means that there are no holes, asymptotes, or jump discontinuities between points a and b. Because the interval contains closed brackets, the graph must also be continuous at points and .
Additionally, if we recall from previous guides, to be differentiable over means that the function is continuous over the interval and that for any point over the interval, exists.
#✏️ Mean Value Theorem: Walkthrough
We can use the Mean Value Theorem to justify conclusions about functions by applying it over an interval. For example:
Let be a differentiable function. The table gives its selected values:
Can we use the Mean Value Theorem to say the equation has a solution where ?
Since it is given that is differentiable, we can apply the Mean Value Theorem (MVT) on the interval . This is what we should write out!
Since is continuous and differentiable on , MVT can be applied. The MVT states that there exists a on such that
so MVT cannot be used to say that has a solution.
#📝 Mean Value Theorem: Practice Problems
Now, it’s time for you to do some practice on your own! 🍀
#❓ Mean Value Theorem: Practice
#Question 1: Mean Value Theorem
Let and let be the number that satisfies the Mean Value Theorem for on the interval .
What is
#Question 2: Mean Value Theorem
Let be a differentiable function. The table gives its selected values:
Can we use the Mean Value Theorem to say the equation has a solution where ?
#✅ Mean Value Theorem: Answers and Solutions
#Question 1: Mean Value Theorem
Since is a polynomial, is continuous on and differentiable on . Therefore, the Mean Value Theorem can be applied. By the Mean Value Theorem, there exists a on such that…
To find , we need to differentiate and find such that
By the quadratic formula, we have .
Since only is in the interval , Great work! Make sure you remember to check if the value(s) you get are in the given interval.
#Question 2: Mean Value Theorem
Since it is given that is differentiable, we can apply the Mean Value Theorem (MVT) on the interval .
The MVT states that there exists a on such that so MVT cannot be used to say that has a solution.
#💫 Closing
The Mean Value Theorem states that for any continuous function on a closed interval, there exists a value c in the interval such that the value of the derivative of the function at c is equal to the average rate of change of the function over the interval. By using this theorem, we can find the mean value of a function on a given interval, which can provide useful information about the behavior of the function. 🧐
Explore more resources

How are we doing?
Give us your feedback and let us know how we can improve





